Nonpartisan Education Review / Essays: Volume 5, Number 2
Access this essay in .pdf format
Discovery learning in math: Exercises versus problems
Barry Garelick*
What is Discovery Learning?
By way of introduction, I am neither mathematician nor mathematics teacher, but I majored in math and have used it throughout my career, especially in the last 17 years as an analyst for the U.S. Environmental Protection Agency. My love of and facility with math is due to good teaching and good textbooks. The teachers I had in primary and secondary school provided explicit instruction and answered students’ questions; they also posed challenging problems that required us to apply what we had learned. The textbooks I used also contained explanations of the material with examples that showed every step of the problem solving process.
I fully expected the same for my daughter, but after seeing what passed for mathematics in her elementary school, I became increasingly distressed over how math is currently taught in many schools.
Optimistically believing that I could make a difference in at least a few students’ lives, I decided to teach math when I retire. I enrolled in education school about two years ago, and have one class and a 15-week student teaching requirement to go. Although I had a fairly good idea of what I was in for with respect to educational theories, I was still dismayed at what I found in my mathematics education courses.
In class after class, I have heard that when students discover material for themselves, they supposedly learn it more deeply than when it is taught directly. Similarly, I have heard that although direct instruction is effective in helping students learn and use algorithms, it is allegedly ineffective in helping students develop mathematical thinking. Throughout these courses, a general belief has prevailed that answering students’ questions and providing explicit instruction are “handing it to the student” and preventing them from “constructing their own knowledge”—to use the appropriate terminology. Overall, however, I have found that there is general confusion about what “discovery learning” actually means. I hope to make clear in this article what it means, and to identify effective and ineffective methods to foster learning through discovery.
To set this in context, it is important to understand an underlying belief espoused in my school of education: i.e., there is a difference between problem solving and exercises. This view holds that “exercises” are what students do when applying algorithms or routines they know and the term can apply even to word problems. Problem solving, which is preferred, occurs when students are not able to apply a mechanical, memorized response, but rather have to figure out what to do in a new situation. Moreover, we future teachers are told that students’ difficulty in solving problems in new contexts is evidence that the use of “mere exercises” or “procedures” is ineffective and they are overused in classrooms.
As someone who learned math largely though mere exercises and who now creatively applies math at work, I have to question this thinking. I believe that students’ difficulty in solving new problems is more likely to be because they do not have the requisite knowledge and/or mastery of skills—not because they were given explicit instruction and homework exercises.
Those who make such a differentiation and champion “true” problem solving espouse a
belief in having students construct their own knowledge by forcing them to make
connections with skills and concepts that they may not have mastered. But, with skills and
concepts still gelling students are not likely to be able to apply them to new and unknown
situations. Nevertheless, the belief prevails that having students work on such problems
fosters a discovery process which the purveyors of this theory view as “authentic work” and
the key to “real learning.”
![]() One teacher with whom I spoke summed up this philosophy with
the following questions: “What happens when students are placed in a totally unfamiliar
situation that requires a more complex solution? Do they know how to generate a procedure?
How do we teach students to apply mathematical thinking in creative ways to solve complex,
novel problems? What happens when we get off the ‘script’?”
One teacher with whom I spoke summed up this philosophy with
the following questions: “What happens when students are placed in a totally unfamiliar
situation that requires a more complex solution? Do they know how to generate a procedure?
How do we teach students to apply mathematical thinking in creative ways to solve complex,
novel problems? What happens when we get off the ‘script’?”
Those are important questions, but I will argue in this article the following points: 1) “Aha” experiences and discoveries can and do occur when students are given explicit instructions as well as when working exercises; and 2) Procedural fluency does not exclude conceptual knowledge—it leads ultimately to conceptual understanding and the two are key for applying mathematics to complex problems.
Let me be clear: I’m not against asking students to discover solutions to novel and challenging problems—the experience can be quite powerful, but only under the right conditions. A quick analogy may be useful here. Suppose a person who knows how to drive automatic transmission cars travels to a city and is forced to rent a car with a standard transmission—stick shift with clutch. The person in charge of rentals gives our hero a basic 15 minute course, but he has no opportunity to practice before heading out. In addition to this lack of skill in driving a standard transmission, the city is new to him, so he needs to rely on a map to get to where he needs to go. The attention he must pay to street names and road signs is now eclipsed by the more immediate task of learning how to operate the vehicle. In fact, he would be wise to take a taxi in order to avoid a serious accident. But now suppose that prior to his trip he is told that he will need to drive a standard transmission because where he is going, rental car companies don’t rent out automatic transmission cars. With proper training and guidance, he can start off on quiet streets to get the feel of how to coordinate clutch with shifting, working up to more challenging situations like stopping and starting on hills. Over time, as he accumulates the necessary knowledge, and practice, he’ll need less and less support and will be able to drive solo. There will still be problems that he has to figure out, like driving in bumper to bumper traffic that requires starting, slowing, downshifting, and so forth, but eventually, he will be able to handle new situations with ease. Now, given the task of driving in a strange city, he will be able to focus all of his attention on navigating through new streets (having already achieved driving mastery of the vehicle that will take him where he needs to go).
Whether in driving, math, or any other undertaking that requires knowledge and skill, the more expertise one accumulates, the more one can depart from the script and successfully take on novel problems. It’s essential that at each step, students have the tools, guidance, and opportunities to practice what they learn. It is also essential that problems be well posed. Open-ended, vague, and/or ill-posed problems do not lend themselves to any particular mathematical approach or solution, nor do they generalize to other, future problems. As a result, the challenge is in figuring out what they mean—not in figuring out the math. Well-posed problems that push students to apply their knowledge to novel situations would do much more to develop their mathematical thinking.
To make this discussion more concrete, let’s take a look at four math problems. The first two are discovery-type problems that the target students do not have the necessary knowledge to solve. The third is an ill-posed problem. The fourth, in contrast, is a well-posed problem that relies upon prior knowledge and is mathematically meaningful.
1. The first problem, intended for 8th graders, comes from a unit on quadratic equations in the
Connected Math Program (CMP).
![]() Although they are just beginning to learn about quadratic
equations, they are expected to somehow discover a rather complex equation. Students are
shown the four figures below, in which dots make up triangle-like shapes, and are told that the
number representing the number of dots in each triangle is called a “triangular number.”
Although they are just beginning to learn about quadratic
equations, they are expected to somehow discover a rather complex equation. Students are
shown the four figures below, in which dots make up triangle-like shapes, and are told that the
number representing the number of dots in each triangle is called a “triangular number.”
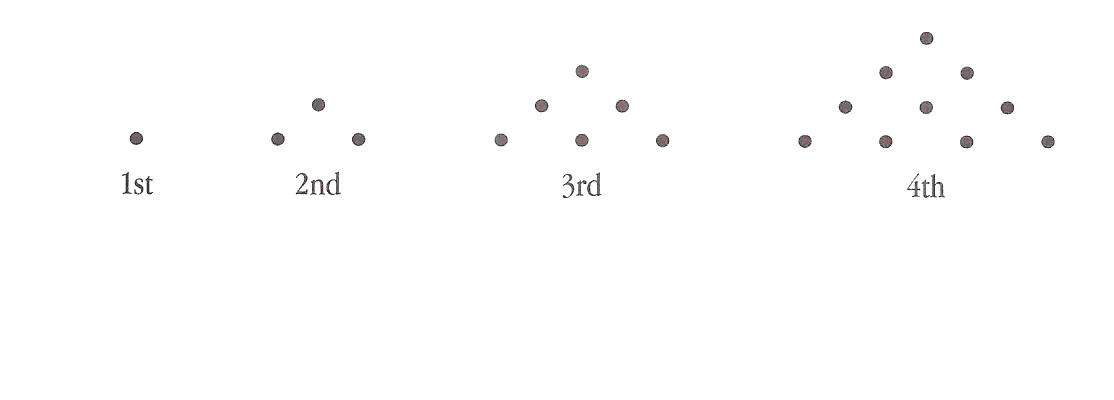
Based on the pattern of these four triangular numbers, students are then asked to find the sixth and tenth triangular number, to make a table of values for the first 10 triangular numbers, and then describe how to use the pattern in the table to find the 11th and 12th triangular numbers and to write an equation for the nth triangular number and explain their reasoning.
This problem is just one of many in an investigation called “Quadratic Patterns of
Change” that would take approximately three to five class periods. According to the
teacher’s guide, the formula for the nth triangular number is
 , but no explanation of
how this is derived is offered until the end of the unit. Also, the fact that the nth triangular
number is the same as the sum of consecutive numbers from 1 to n is not clarified until the
end of the unit.
, but no explanation of
how this is derived is offered until the end of the unit. Also, the fact that the nth triangular
number is the same as the sum of consecutive numbers from 1 to n is not clarified until the
end of the unit.
Students are next presented with a series of problems involving handshakes: What is the number of handshakes between two teams with 5 players on each team, with n players? What if one team has one more player than the other? What is the number of handshakes within the same team? The teacher's guide suggests some methods to try with students to extract that last one, but by then students are likely to be mightily confused—particularly those who still haven’t made the discovery that triangular numbers are the same as the sum of consecutive numbers.
Students are then presented with even more problems related to triangular numbers. Finally a question brings home the point that triangular numbers are the same as the sum of consecutive numbers 1 through n. Right after that students are shown a method for deriving the equation. At this point, students might well be asking, “Why didn’t you show us this in the first place?” Although this is supposed to be a unit on quadratic equations, students are given so much to explore that the systematic development of a method to solve such equations is lost. The activities are the main course and quadratic equations end up in a side dish.
2. The second discovery-type problem comes from the first-year textbook of the Interactive
Math Program series (IMP)
![]() and is given to students who have just started algebra. These
students have had limited exposure to systems of linear equations—for example, two
equations with two unknowns.
and is given to students who have just started algebra. These
students have had limited exposure to systems of linear equations—for example, two
equations with two unknowns.
“You have five bales of hay. For some reason, instead of being weighed individually, they were weighed in all possible combinations of two: bales 1 and 2, bales 1 and 3, bales 1 and 4, bales 1 and 5, bales 2 and 3, bales 2 and 4, and so on. The weights of each of these combinations were written down and arranged in numerical order, without keeping track of which weight matched which pair of bales. The weights in kilograms were 80, 82, 83, 84, 85, 86, 87, 88, 90, and 91. Your initial task is to find out how much each bale weights. There may be more than one possible solution; if this is so, find out what all of the solutions are and explain how you know.”
Students who are just beginning algebra do not have the prerequisites to solve this problem efficiently. They would probably have to use “guess and check,” a method that might result in the right answer, but that is not likely to deepen students’ understanding. A key observation necessary to solve the problem efficiently is that no two bales are equal in weight because no two of the sums provided in the problem are equal. Such a relationship is not easy for beginning algebra students to see, and is only one of several different kinds of reasoning required to solve such a problem.
How would beginning algebra students with little foundation in systems of linear
equations and mathematical reasoning feel when confronted with such a problem? David
Klein, a mathematics professor from California State University at Northridge, commenting
on this problem, said, “It is an annoying problem and has little educational value. If I had
been given such problems at that age, I think that I would have hated math.”
![]() Why such
strong words? Because it is unlikely that guess-and-check will provide any insight that can
be transferred to other problems or result in a deeper understanding of mathematics.
Meanwhile, the foundational knowledge that comes with mastery of different types of
algebraic problems over time has not been learned.
Why such
strong words? Because it is unlikely that guess-and-check will provide any insight that can
be transferred to other problems or result in a deeper understanding of mathematics.
Meanwhile, the foundational knowledge that comes with mastery of different types of
algebraic problems over time has not been learned.
3. Our third example is not a discovery-type problem like those above. Rather, it is an ill-posed problem that can be interpreted many ways and, as a result, is not educational. This
problem comes from the “Ten-Minute Math” section of a teacher’s guide for TERC’s
“Investigations in Number, Data, and Space” for fourth grade.
![]() In this particular activity,
students decompose numbers in an exercise that is ultimately designed to get students to
think beyond place value. The guide explains that decomposing numbers “is more than just
naming the number in each place. It includes understanding, for example that while 335 is 3
hundreds, 3 tens, and 5 ones, it is also 2 hundreds, 13 tens, and 5 ones”
In this particular activity,
students decompose numbers in an exercise that is ultimately designed to get students to
think beyond place value. The guide explains that decomposing numbers “is more than just
naming the number in each place. It includes understanding, for example that while 335 is 3
hundreds, 3 tens, and 5 ones, it is also 2 hundreds, 13 tens, and 5 ones”
![]() and then proceeds
with the following instructions (for the teacher) and questions:
and then proceeds
with the following instructions (for the teacher) and questions:
Step 1 Write or say a number. Write a number on the board (or say it and have students write it.) For example:
1,835
Step 2 Ask: "How many groups of _______ (10, 100, 1,000, etc.) are in the number? For example, ask students how many groups of hundreds are in 1,835. If students think that eight is the only answer, ask them to consider a context such as money.
If this were money, how many hundred dollar bills would we have if we had $1,835?
Establish with students that there are 18 hundreds in 1,835.
The problem is poorly worded and constructed given the answer the authors seek, and I feel sorry for the dedicated student who tries to make sense of it. Students who have an understanding of place value and see the 8 in 1,835 as representing 8 hundreds are now confronted with conflicting information: there is more than one answer, and 8 is not the answer being sought. They are then guided to make a “discovery” that there are 18 hundreds by making a connection that if there are 18 hundred dollar bills contained in $1,835, then there are 18 hundreds in 1,835. Those are two different statements. Worse, the latter is mathematically incorrect in the context of the question asked. Since the previous “Ten-Minute Math” focused on decimals, students may reason—correctly—that although there are 18 hundred dollar bills in $1,835, there are actually 18.35 hundreds contained in 1,835. Asking how many hundreds are in 1,835 is a division problem (1,835/100), but the activity calls it a place value problem, and the result is an incorrect answer. If students are not already profoundly confused by all this, they will be soon: the activity then asks them to “make up five different combinations of place values [sic] that equal 1,835: 15 hundreds + 33 tens + 5 ones; 16 hundreds + 23 tens + 5 ones; and so on.”
While the problem may result in students thinking of different answers, it does not encourage mathematical thinking, does not push students to further their knowledge of mathematics, it incorrectly characterizes place value and in so doing, it confuses more than enlightens.
4. Our fourth example offers a sharp contrast to the other three. This problem comes from the
fourth grade textbook in the series called Primary Mathematics from Singapore.
![]() It is well
posed and requires students to apply their prior knowledge.
It is well
posed and requires students to apply their prior knowledge.
“What is the value of the digit 8 in each of the following?
a) 72,845 b) 80,375 c) 901,982 d) 810,034 e) 9,648,000 f) 8,162,000”
Students cannot escape the lesson about place value since they cannot simply note where the 8s are, they must know what the various positions of the 8s mean. Preceding this problem in the Singapore text are other problems that introduce the concept of a number being a representation of the sum of smaller components of that number by virtue of place value; i.e. 1,269 can be expressed as 1,000 + 200 + 60 + 9.
Similarly, students are asked to express written out numbers, such as ninety thousand ninety, using numerals in the standard form (i.e., 90,090). They are also asked to write numbers in numeral form, such as 805,620, in words.
In short, students are asked no ambiguous questions, and the underlying concept of place
value is indicated clearly via examples that can be applied directly to problems. By the time
students reach the problem asking for the value of “8” in the various numbers, they have a
working knowledge of what the numbers in various positions represent. This problem pushes
them to apply that knowledge, thereby revealing any confusion they may have and also
providing enough guidance for them to see that the position of the number dictates its value.
![]()
Advocates of complex problems that get students “off the script” may think this problem is not challenging enough. After all, any discovery students make is inherent in the presentation of the problem and the solution clearly comes from work that the students have just completed. But as anyone recalls from the early days of having to learn something new, it feels a whole lot different answering questions on your own, even after having received the explanation. In fact, such experience constitutes discovery. So I have to ask, what is wrong with acquiring incremental amounts of knowledge through well-posed problems? It is, after all, much more efficient than discovery-type problems that require Herculean sense-making efforts and leave most floundering for a solution, without a clear sense of whether they are right or wrong.
Instruction that uses such problems isn’t “handing it to the student.” To the contrary, it’s
providing the support and guidance that students need to grow. I see it as a staging; a way to
get students to apply easier problems to solve harder ones, and a way for procedural fluency
to lead to understanding.
![]() The key is for problems to be carefully sequenced such that they
incrementally increase in difficulty and require students to use their knowledge in new
ways—and that’s the key to making a meaningful discovery.
The key is for problems to be carefully sequenced such that they
incrementally increase in difficulty and require students to use their knowledge in new
ways—and that’s the key to making a meaningful discovery.
Making Meaningful Discoveries
To better explain the effectiveness (and efficiency) of carefully sequenced, well-posed problems it is important to understand the process of “scaffolding”. This is the process of extending previously learned material to slightly more difficult problems. When done properly—whether in a set of homework problems or in a classroom—it is an extremely effective method to bring about meaningful discovery. I have had the pleasure of observing a teacher who does this very well. Let me tell you about one of her lessons.
The lesson I’d like to share with you is from the teacher’s honors geometry class. Having already taught the students the basics of trigonometry and how to use it to find the lengths of the sides of a right triangle in a previous lesson, she drew the following figure on the board:

She asked the class to find the perimeter of the triangle (which is not a right triangle) given the two angles, and length of one side as shown. She told them that they had all the knowledge necessary to solve it.
The key to solving this problem is to draw in the altitude and then, using trigonometry, solve for the lengths of various sides.
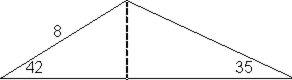
Some students drew in the altitude and solved it. Others were stymied and said they couldn’t solve it “because it isn’t a right triangle.” The teacher provided a hint: “Well maybe you have to create a right triangle.” They thought about this and understood that they needed to draw the altitude. I asked one girl who had drawn in the altitude without any hint from the teacher her how she knew what to do. She explained that drawing in the altitude of a triangle was something she did automatically any time she had a triangle—she didn’t really know why. In this case it helped her to solve the problem—something I’ll come back to in the next section on critical thinking.
Algorithmic Procedures and the Development of Critical Thinking
Mathematics demands mastery of foundational steps in order to build upon them. As such, it is relentlessly linear. Without such mastery or foundation students will not be prepared to solve new and complex problems. Yet some practitioners believe that the above example is “inauthentic work”—mere exercises—and as such, does not lead students to learn how to do the “authentic work” of solving problems.
In fact, the application of learned and mastered material in new, off-the-script context
does not happen immediately—nor is it brought about by giving students problems which
they‘re not equipped to solve. Daniel Willingham, a cognitive scientist who teaches at the
University of Virginia, maintains that it takes time and effort for knowledge to accumulate to
the point that connections between learned material and new and difficult problems can be
made.
![]() Willingham refers to the difficulty that novices have with thinking critically as
“inflexible thinking,” which he characterizes as perfectly normal and to be expected among
students. Specifically, the critical thinking that allows for the application of prior knowledge
to new, unfamiliar type problems requires recognition of an underlying principal that can be
used to solve the problem. For example, the following two problems are based on the same
underlying principle—rate—and in fact the same equation is used to solve both of them:
Willingham refers to the difficulty that novices have with thinking critically as
“inflexible thinking,” which he characterizes as perfectly normal and to be expected among
students. Specifically, the critical thinking that allows for the application of prior knowledge
to new, unfamiliar type problems requires recognition of an underlying principal that can be
used to solve the problem. For example, the following two problems are based on the same
underlying principle—rate—and in fact the same equation is used to solve both of them:
1) John can mow the lawn in 20 minutes while his brother Bob can mow the same lawn in 30 minutes. If both mow at a constant rate, how long does it take for both of them to mow the lawn together?
2) It takes John 20 minutes to walk to school from home, while it takes his sister 30 minutes, both walking at constant rates of speed. John starts walking from school to home at the same time that his sister starts walking from home to school. How long will it take for them to meet?
For the first problem the rate is the portion of the job completed per minute. Specifically,
John's rate is 1/20 of the job per minute and Bob’s rate is 1/30 of the job per minute. The
amount accomplished in X minutes is
for John and
for Bob. Therefore, the
portion of the lawn mowed (or job completed) in X minutes by both John and Bob working
together is
. In setting it up this way, we are adding up their accomplishments
in terms of how much of the job is completed in X minutes. Since the problem asks how
long it takes to mow the lawn, we are interested in exactly one job, and want to find what
value of X is needed to get this done. To do this, we set the above expression equal to 1 and
solve for X:

The second problem is solved using the same reasoning as the first. The key to solving it is to see the connection between distance and time, and that the rate is the portion of the total distance walked per minute. In one minute John walks 1/20 of the way between school and home, while his sister walks 1/30 of the way. The solution follows as described above for the lawn mowing problem, and results in the same equation.
Beginning algebra students may understand how to solve the first problem but may not make the connection that the same concept of rate underlies the second problem as well In fact, as Willingham explains, it is unlikely that students will make such connections readily until they have developed true expertise. Only experts see beyond the surface level of a problem to its deeper structure.
So how do you teach students to make such connections; i.e., to think critically? Is it a failure of the math program—or the teacher—if they do not? Willingham argues that understanding the deep structures of a discipline such as mathematics is an important goal of education, “but if students fall short of this, it certainly doesn’t mean that they have acquired mere rote knowledge and are little better than parrots.” Rather, they are making the small steps necessary to develop better mathematical thinking. Simply put, no one leaps directly from novice to expert.
While there is no direct path to learning the thinking skills necessary to apply one’s knowledge and skills to unfamiliar territory, Willingham argues that one way to build a path from inflexible to flexible thinking is to use examples. This approach could be used for rate problems such as the two problems just described. In fact, this is what was done in the two well crafted lessons I observed. Students extended their knowledge along scaffolding built from examples—examples that fit on the underlying structure.
Although it does not necessarily happen automatically, thinking becomes more flexible as more knowledge and experience are acquired. Think of the girl in the high school geometry class who solved the triangle problem. What had become a mechanical or algorithmic habit for her—drawing in the altitudes of triangles—ultimately led to the solution. Many problems in mathematics involve evaluating their form through algebraic manipulation, or in the case of geometric figures, adding supplementary lines. Such analysis leads to insights about a problem’s underlying structure. The girl’s habit, which some might consider algorithmic thinking (and therefore “inauthentic”) was part and parcel of her flexibility in thinking and applying a previously learned principle in a novel way to a new problem.
Students given well-defined problems that draw upon prior knowledge, as described in this article, are doing much more than simply memorizing algorithmic procedures. They are developing the procedural fluency and understanding that are so essential to mathematics; and they are developing the habits of mind that will continue to serve them well in more advanced, college level mathematics courses. Poorly-posed problems with multiple “right” answers turn mathematics into a frustrating guessing game. Similarly, problems for which students are expected to discover what they need to know in the process of solving it do little more than confuse. But well-posed problems that lead students in manageable steps not only provide them the confidence and ability to succeed in math, they also reveal the logical, hierarchical nature of this powerful and rewarding discipline.
*Barry Garelick is an analyst for the federal government and lives in Fairfax County, Virginia. He is a national advisor to NYC HOLD, an education advocacy organization that addresses mathematics education in schools throughout the United States.
Citation: Garelick, B. (2009).Discovery learning in math: Exercises versus problems. Nonpartisan Education Review / Essays, 5(2). Retrieved [date] from http://www.nonpartisaneducation.org/Review/Essays/v5n2.htm
Access this essay in .pdf format